All published articles of this journal are available on ScienceDirect.
Effect of Sample Pooling on the Cycle Threshold Value in RT-PCR Testing for COVID-19 and Logistic Savings
Abstract
Background:
This ongoing coronavirus disease 2019 (COVID-19) pandemic is a great challenge for our health-care systems and their infrastructure. Diagnostic confirmation of infected individuals based on RT-PCR is important for the containment of viral spread because, despite high viral loads, the infection can be asymptomatic. For overcoming the issue of limited supplies in low socio-economic countries like ours, pooling of specimens was proposed as a method to screen a large number of patients. To analyse the effect of pooling of samples on the sensitivity of RT-PCR, we compared Cycle threshold (Ct) values of pools with those of the deconvoluted (individual) samples.
Materials and Methods:
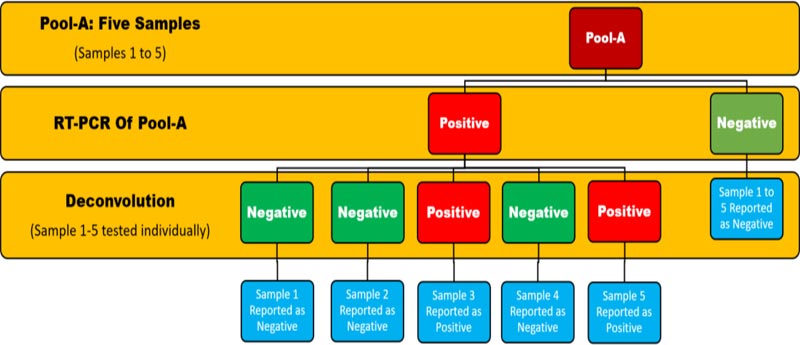
From March 2020 to October 2020, we performed COVID-19 testing by RT-PCR on samples from areas with varying prevalence of COVID-19 referred to VRDL, JNMCH, Aligarh. For circumventing the limited availability of RNA extraction and PCR reagents, pool testing was started. 5 samples were pooled together, and these pools were tested by RT-PCR. Negative pools were reported as negative, whereas positive pools were deconvoluted, and each sample was tested individually. In this study, 408 pools comprising of 2040 samples were analysed.
Observations and Results:
In our study, a ΔCt value of 0.96 (i.e., an increase in Ct value in the pooled sample as compared to individual sample) was found that shows a slight loss of PCR sensitivity in pooled samples, which is relatively small compared to the inherent clinical sensitivity of the standard assay. But the cost-effectiveness and the ability to reserve resources are considerably high.
Conclusion:
As pooling of samples is a cost-effective way for COVID--19 testing, the slight loss in the sensitivity of RT-PCR can be overcome by considering the Ct cut-off value for positive pools slightly above the kit cut off value to circumvent the dilution effect.
1. INTRODUCTION
1.1. Novel Coronavirus
This ongoing coronavirus disease 2019 (COVID-19) pan- demic is a great challenge for our health-care systems and their infrastructure. It has highlighted the need for early diagnosis of the disease for the better containment of this outbreak. Diag- nostic confirmation of infected individuals based on RT-PCR is important for containing viral spread because, despite high viral loads, the infection can be asymptomatic. Sufficient molecular diagnostic capacity is important for public health interventions, such as case detection and isolation [1]. The rapid diagnosis of COVID-19 in both symptomatic and asymptomatic patients can shed light on transmission patterns and facilitate contact tracing [2, 3]. To date, countries that were able to screen patients swiftly have fared better in containing the COVID-19 outbreak and suppressing the mortality rate associated with the disease [3].
Protocols for RNA RT-PCR testing of Severe Acute Respiratory Syndrome Coronavirus 2 (SARS-CoV-2) became available early in the pandemic, yet the infrastructure of testing laboratories is stretched and, in some areas, it is overwhelmed [4]. Later on, the testing for SARS-CoV-2 has been limited due to the considerable strain on global supply chains for PCR kits, reagents, personal protective equipment, and other consum- ables [2, 5].
1.2. Economic approach for Testing Covid-19
For overcoming the issue of limited supplies in low socio-economic countries like ours, pooling of specimens was proposed as a method to screen a large number of patients for infection. It typically involves combining multiple patient specimens into a single test sample and then testing these pooled samples. This approach has the advantage of cost-effectiveness and speedy reporting. It was also used to retrospectively screen for COVID-19 in specimens that were negative for common respiratory viruses earlier in the course of the pandemic in the United States [6]. Specimen pooling has also been used in screening efforts for several other infectious diseases, including donated blood samples for HIV [7-10].
It is unclear how pooling biological samples would affect the sensitivity and the false-negative rate of Polymerase Chain Reaction (PCR) assays. To analyse the effect of pooling of samples on the sensitivity of RT-PCR, we compared Cycle threshold (Ct) values of pools that tested positive with Ct values of individual samples that tested positive.
2. MATERIALS AND METHODS
Between March and October 2020, we performed COVID-19 testing by RT-PCR from areas with varying prevalence of COVID-19 referred to VRDL, JNMCH, Aligarh. In the initial days, samples were being tested individually, but due to the increasing workload of testing pool testing was started to circumvent the limited availability of RNA extraction reagents. Five samples were pooled together, and then these pools were tested by RT-PCR. Negative pools were reported as negative, whereas positive pools were deconvoluted, and each sample was tested individually (Fig. 1). In this study, we studied data of 408 pools comprising of 2040 samples ; CT values of pools were compared and analysed with respect to deconvoluted samples.
2.1. Calculation of Delta Ct (ΔCt): Sensitivity of Pool Testing
The delta Ct value (ΔCt) was defined as the absolute change in Ct value when the pooled sample was tested compared to when the positive samples were tested individually. A positive ΔCt value (i.e., an increase in Ct value in the pooled sample) represented the loss of PCR sensitivity attributable to sample pooling.
2.2. Calculation of the Effect of SARS-CoV-2 Prevalence, Pool Size on Efficiency and Logistics
The effect of SARS-CoV-2 prevalence, pool size on efficiency and logistics was derived by the web-based application, utilizing the R statistical software environment available at: http://www.chrisbilder.com/shiny/.
3. RESULTS AND DISCUSSION
3.1. Sensitivity of Pool Testing
The mean Ct of the positive sample in each pool, the mean Ct of the pooled sample, and the mean ΔCt are shown in Table 1.
| Pool Size | Mean Ct of Positive Samples in the Pool (Standard Deviation, Range) | Mean Ct of the Pool (Standard Deviation, Range) |
Mean ΔCt (Range) |
|---|---|---|---|
| 5 samples | 26.56 (3.93, 14 to 34) | 27.51 (4.33, 15 to 37) | 0.956 (-8 to +12) |
In our study, a ΔCt value of 0.96 (i.e., an increase in Ct value in the pooled sample as compared to individual sample) was found, that shows a slight loss of PCR sensitivity in pooled samples which is relatively small compared to the inherent clinical sensitivity of the standard assay. But the cost-effectiveness and the ability to reserve resources are considerably high.
3.2. Relation between Ct Values of the Pool versus mean of Ct Values of Individual Positive Samples
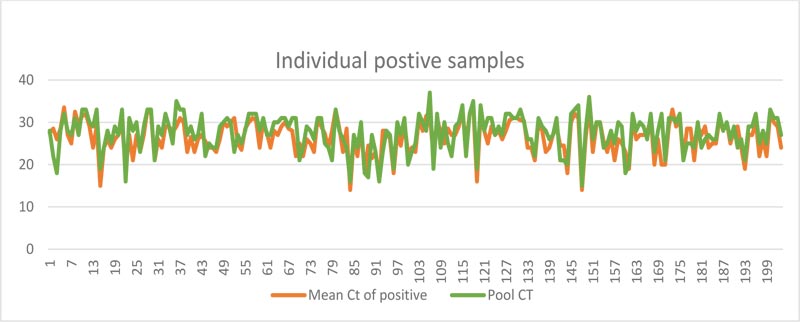
As expected, the Ct values of the pool showed a near-perfect linear relation with the mean of Ct Values of individual positive samples in the pool (Fig. 2)
3.3. Effect of SARS-CoV-2 Prevalence and Pooling on the Efficiency and Logistics
Table 2 and Fig. (3) shows the number of extraction and PCR reactions required for testing of samples in the pool size of 5 based on disease prevalence (inclusive of initial pool testing and deconvolution of positive pools).
| Prevalence of COVID-19 (%) | Five-sample Pools | ||
|---|---|---|---|
| Number of Tests Per Sample | Number of Tests for 1000 Samples |
Saving of the Reagents and Test Cost (Percentage) |
|
| 0.5 | 0.23 | 230 | 77 |
| 5 | 0.43 | 430 | 57 |
| 10 | 0.61 | 610 | 39 |
| 15 | 0.76 | 760 | 24 |
| 20 | 0.87 | 870 | 13 |

3.4. Determining the Optimal Pool Size for Effective Testing
As the PCR-positivity is a function of the net viral load in the pooled sample, we understand
that the success of the pooling strategy as a sensitive screening tool would be dependent on the number of infected patients in the pool and the viral load in the individual samples. While pooled sample testing offers the advantage of cost-effectiveness and timely reporting, the strategy becomes less useful in communities where the prevalence of the disease is high. With increased prevalence, the number of positive pools is likely to increase, and more pools are to be deconvoluted for identifying the positive individuals negating the advantages, such as cost-effectiveness and time-saving. Furthermore, with the increase in the prevalence of the disease, the negative predictive value (NPV) of the diagnostic test for the disease decreases, leading to higher false-negative results. Based on this premise, national advisories have suggested testing of 5 sample pools for COVID-19 in communities with the prevalence of up to 5% [11].
3.5. Advantages of Pooling Strategy with Respect to Logistics
To understand the advantages of a pooling approach, consider a laboratory receiving N = 200 samples; 40 pools will be made out of it, and as the prevalence is 5%, therefore, 10/200 samples will be positive as a best-case scenario, i.e., we can have 4 pools positive and 16 pools negative and the total PCR reagent used to test 200 samples will be 60. In the worst-case scenario, 10 pools will be positive thus, the total reagent used will be 90. Therefore, taking the mean value as 75, we propose that for 200 samples in pooled testing, we require 75 test reagents, thus saving 62.5% reagents. The present study is concordant with the findings of Abdal Hamid et al., who reported that pool testing is effective in saving resources in the population having a prevalence less than 5% [12].
In this study, A ΔCt value of 0.96 (i.e., an increase in Ct value in the pooled sample as compared to individual sample) was found that shows a slight loss of PCR sensitivity in pooled samples which is relatively small compared to the inherent clinical sensitivity of the standard assay [13]. Our ΔCt value is quite less (0.9) as compared to others like Garg et al., who found a mean difference of 5.0 [14].
Duringn the COVID-19 pandemic, pooling of samples started as a method for testing large no. of samples to save resources which later became a highly practiced phenomenon in low socio-economic countries. A recent study by Lohse showed that over a range of pool sizes, from 4 to 30 samples per pool, Ct values of positive pools fall between 22 and 29 for the envelope protein gene (E-gene) assay and between 21 and 29 for the spike protein gene (S-gene) assay. Ct values were lower in retested deconvoluted positive samples. The Ct values for both E-gene and S-gene assays in pools and deconvoluted positive samples were below 30 and can easily be categorized as positive. Ct value differences between pooled tests and deconvoluted positive samples (Ct pool − Ct positive sample) were in the range of up to 5 [15]. It can be explained by the fact that for each two-fold dilution, Ct value increased by 1.24 [16]. Thus, in pooled samples, graphs should be analysed for the sigmoid curve even beyond Ct value 40, and in the case of the appearance of any graph, the RT-PCR should be repeated with deconvoluted samples, and by following the above-mentioned precautions, we can pick maximum possible positive cases.
CONCLUSION
During a rapidly changing pandemic, testing strategies will need to adapt to increase the potential to test maximum possible samples with low resources, in the least possible time with a quality result. This study showed that pooling is an effective approach to expand the impact of limited test resources and reagents during specific stages of an infectious disease outbreak as it provides quality reports in less time, cost, and manpower. As pooling of samples is a cost-effective way for COVID--19 testing, the slight loss in the sensitivity of PCR can be overcome by considering the Ct cut-off value for positive pools slightly above the kit cut-off value to circumvent the dilution effect. Also, group testing of pooled specimens requires the use of highly sensitive assays to avoid missing low positive samples. In addition, the impact of different extraction methods on the recovery of RNA and overall test sensitivity needs further evaluation.
ETHICS APPROVAL AND CONSENT TO PARTICIPATE
Not applicable.
HUMAN AND ANIMAL RIGHTS
Not applicable.
CONSENT FOR PUBLICATION
Not applicable.
AVAILABILITY OF DATA AND MATERIALS
The authors confirm that the data supporting findings of this study are available within the article.
FUNDING
None.
CONFLICT OF INTEREST
The authors declare no conflict of interest, financial or otherwise.
ACKNOWLEDGEMENTS
We acknowledge the support of Dr. Syed Ghazanfar Ali, Dr. Islam, Mr. Zeeshan, Mr. Shah Alam, Mr. Farhan, Mrs. Shibli Javed and Mr. Sanaullah for their tireless efforts in RT-PCR testing, without which this study would not have been possible.


